when i first heard it i thought it sounded like mainstream pop and didnt like it, but after hearing it a few more times, i starting to like it a lot, maybe not to the level of emptiness machine, still it good a cool rhythm and it vibes
proprietary, btw
Hint:
spoiler
Try out the following tasks before going for the big one
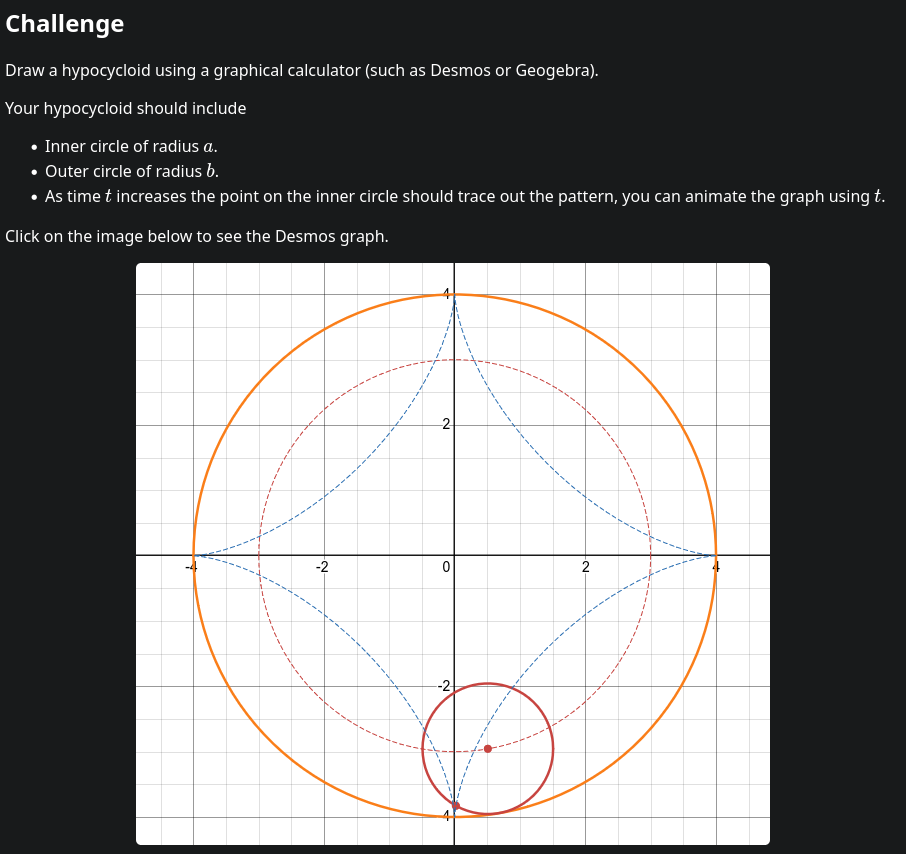
- Draw a circle of radius
a. - Animate a point on circle
a, let that be your rotational speed. - Animate a circle rolling horizontally (along the
xaxis) at your rotational speed. - Animate a point on that horizontally rolling circle.
You should now have an idea on how to draw a hypocycloid.
Hint
spoiler
If you are studying the algorithm, you are doing it wrong
Solution: https://gmtex.siri.sh/fs/1/School/Extra/Maths/Qotd%20solutions/2024-08-04_extended-euclid.html
spoiler

Hint:
spoiler
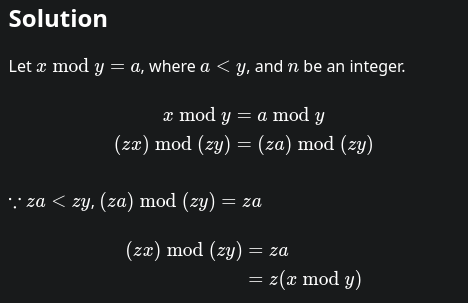
Let x mod y = a
Solution: https://gmtex.siri.sh/fs/1/School/Extra/Maths/Qotd%20solutions/2024-08-01_multiple-of-modulus.html
spoiler
Hint:
spoiler
The size of a set is the number of possible values that an element can take.
because I have never heard of this argument before, ever. most media's stance on politics is "their party bad our party good", but the "all the parties are pretty hypocritical" argument has never been explored properly, because its depressing and nobody likes it.
yup thats the intended solution, im not really familiar with taylor series yet, but maybe for a person who knows taylor series would be able to see it right away
Hint
spoiler
The solution I have in mind is related to the Taylor series
Hint 2
spoiler
It converges to -ln(2), but why
Solution:
i main zathura, but okular is a good one as well
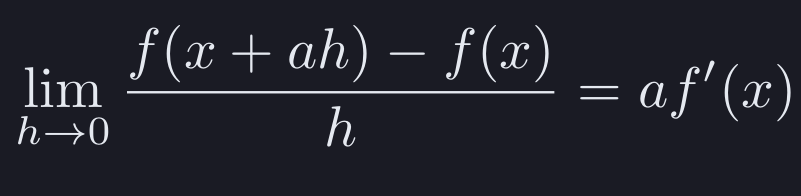











I've seen some people talking about the reason this song being pop is that all albums must have a radio song where almost everyone in the general public would accept. So my personal take while this song diverges from the classic LP style and the 2 previous songs, it doesn't really count towards the theme of the upcoming album.
I hope this song is just a show of "we can do this style" rather than "we will be doikg this style from now on", because I can already name a handful of pop songs with a similar style, and it kinda loses the point of LP.